Cosmos


COSMOS - The SAO Encyclopedia of Astronomy › S

Schwarzschild Lens

The Schwarzschild lens model is the simplest gravitational lens model. It treats the lensing object as a point mass in the lens plane, and always produces two images (although one image may be highly demagnified).

For the Schwarzschild lens, the deflection angle, $ \hat{\vec{\alpha}}(\vec\xi) $, is:

$ \hat{\vec{\alpha}}(\vec\xi) = \frac{4GM}{c^2|\vec\xi|^2}\vec\xi $

where M is the lens mass, $ \vec\xi $ is the impact parameter in the lens plane, G is the gravitational constant and c is the speed of light. The closer a light ray passes to the lens, the greater the deflection.

If we use the dimensionless gravitational lens equation:

y = x – α(x)

relating positions in the source plane, y and lens plane x, the deflection angle for the Schwarzschild lens reduces to:

α(x) = 1/x

This equation can be inverted to give a simple quadratic equation, and hence $ x_\pm $ (the two image locations) as a function of y. We now have enough information to determine:

  • The location of the source, if we know the location of one or both of the images, using y = x – 1/x; and
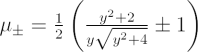  • The location of both images, if we know the location of the source, using $ x_\pm = \frac12\left[y\pm \sqrt{y^2+4}\right] $. The corresponding gravitational magnifications of the two images are $ \mu_\pm = \frac12\left(\frac{y^2+2}{y\sqrt{y^2+4}}\pm 1 \right) $.

For a point source which is directly in line with the observer and a Schwarzschild lens, the image will be a ring with radius:

$ \xi_E = \sqrt{\frac{4GM}{c^2}\frac{D_{OL}D_{LS}}{D_{OS}}} $

called the Einstein ring or Einstein radius. The distances, Dij, are angular diameter distances between the [O]bserver, [L]ens and [S]ource planes.


Study Astronomy Online at Swinburne University
All material is © Swinburne University of Technology except where indicated.