Angular Velocity
For rotational motion about a point or axis, angular velocity is the rate of change of the angular position with time, or in other words the derivative of the angular position with time:
| where | θ = the angular position | |
| t = time |
|---|
The direction of the angular velocity vector is perpendicular to the plane of rotation as given by the right-hand rule. The angular velocity is expressed in units of [angular distance/time], often radians per second.
For an object moving in a curved path it can be useful to describe the motion using both angular and linear velocities. Using a fundamental relation for circular geometry:
| |
|||
| where | θ = angle |
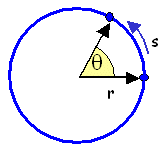
|
|
| s = arc subtended by θ | |||
|---|---|---|---|
| r = radius of the circle |
The magnitudes of the linear and angular velocities are related by:
| where | v = linear velocity | |
| r = distance from the axis of rotation | ||
|---|---|---|
| ω = angular velocity |
Note that we are not using vector notation in this expression, rather it is the magnitude of the velocities that follow the relation.
Study Astronomy Online at Swinburne University
All material is © Swinburne University of Technology except where indicated.

