SAO Maths Primer

SAO Maths Primer |
 |
Mathematics Primer: Integrals
integral
Usually, "to integrate" has two meanings. Firstly, it has a non-technical definition, "to indicate the whole of; to give the sum or total of" (Webster). Mathematically, this meaning is represented by finding areas bounded by curves, volumes of solids, lengths of curves, etc. An integral is a mathematical object which can be interpreted as an area or a generalisation of area. We will discuss this interpretation later.
Another mathematical meaning of "to integrate" is to find a function whose derivative is given. Other words for integral include antiderivative or inverse derivative. Formally, a function y = F(x)
is a called a solution of the differential equation:
Integral as area under a curve
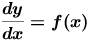
if over the range a < x < b, F(x) is differentiable and
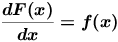
We can also say that in these circumstances, F(x) is an integral of f(x) with respect to x .
Further, if y = F(x) is any solution then all solutions are contained in the formula
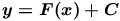
where C is an arbitary constant. This is indicated by the following expression:
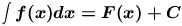 |
 |
We may interpret this by thinking of the symbol
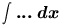
as meaning "integral, with respect to x , of ...". This symbol may then be interpreted as the inverse of the symbol
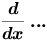
As an example we display an equation describing the total luminosity of a star.
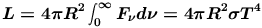 |
 |
This equation describes the luminosity of a star as a function of the integral of the flux emitted at the star's surface. There are limits in frequency given, 0 and infinity, over which the flux is integrated (i.e. summed), to give total flux in all frequencies.
|
From the geometrical point of view the integral of a function f(x) between points x=a and x=b , is equivalent to the area delimited by the curve f(x), the x axis and the vertical lines at x=a and x=b . Then the area shaded in blue in the picture is:
|
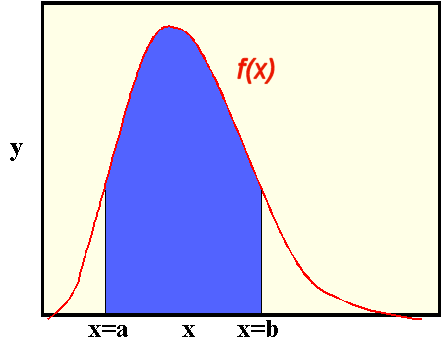 |
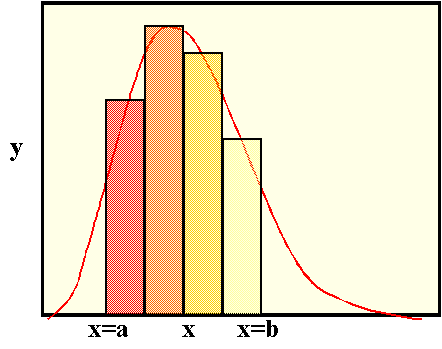
To illustrate how the concept of integral as area under a curve arises, we first consider that the area under the curve can be approximated by splitting it into rectangles of width and height given by:
|
So, the area under the curve can be approximated by the sum of the areas of all of the N rectangles:
|
 |
Now we can make the approximation better by making the rectangles narrower (i.e. 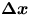 smaller), and therefore increasing the number of them (i.e. larger N ).
smaller), and therefore increasing the number of them (i.e. larger N ).
|
The concept of integral as the area under the curve arises when we consider the limit in which the width of the rectangles approaches zero (or equivalently the limit toward an infinite number of rectangles). This summation of areas in the limit of infinite rectangles is called the integral of f(x) from a to b .
|
 |
Example
|
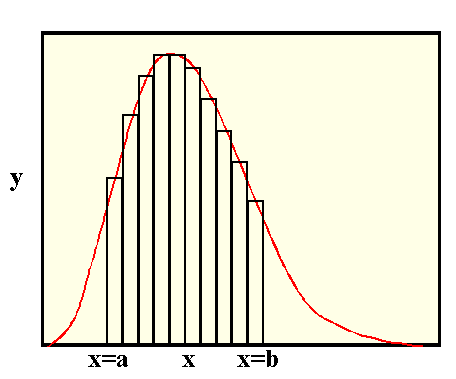
Consider the function f(x)=mx (this is just a line of slope m) and let's calculate the area under the line between points x=a and x=b . Geometrically we can just calculate the area of the triangle as shown in the picture. This is:
If we calculate the integral of the function f(x)=mx between x=a and x=b we can see that it is equal to the area under the curve:
|
 |
Eric Weisstein's world of Mathematics mathworld.wolfram.com
S.O.S. Mathematics www.sosmath.com
Return to Maths Primer page
Back to the Swinburne Astronomy Online Home Page
©
Swinburne
Copyright and disclaimer information
Maintained by: Rebecca Allen
(rebeccaallen@swin.edu.au)
Authorised by: Prof. Jean Brodie (jbrodie@swin.edu.au)
Monday, 19-Nov-2007 11:19:37 AEDT